North American AstroPhysical Observatory (NAAPO) Cosmic Search: Issue 4 (Volume 1 Number 4; Fall (Oct., Nov., Dec.) 1979) [Article in magazine started on page 25]

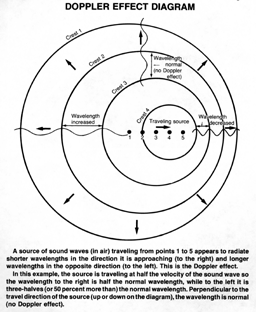
ABCs of Space By: John Kraus A. The Doppler Effect (Wavelengths are relative. The Red Shift) When a rapidly moving vehicle passes with its horn sounding, there is an abrupt decrease in the pitch of the horn. This is the Doppler effect, first described by Christian Johann Doppler of Vienna in 1842. A decrease in pitch (or frequency) corresponds to an increase in wavelength. Thus, a receding object has a longer wavelength (or lower frequency) than when it is approaching. The effect can be observed for sound waves, water waves and electromagnetic waves (light and radio waves). Referring to the Doppler Effect Diagram, suppose a source of waves is moving to the right. When it was at position 1, it sent out the crest of a wave, by the time the source reached position 2 it sent out another crest and so on. By the time it reached position 5, the previously emitted crests had expanded to the circles shown. The wavelength (or crest to crest) distance is less in the direction toward which the source is traveling (to the right) and greater in the direction from which it is receding (left). This is the Doppler effect. Perpendicular to the direction the source is traveling, the wavelength is normal (no Doppler effect). Suppose that the source is sending out a sound wave in air at middle-C. Then an observer to the right hears high-C (wavelength halved), while an observer to the left hears F-below-middle-C (wavelength 50 percent longer). As explained in ABCs for the Summer 1979 issue, chemical elements have characteristic spectral lines at specific optical wavelengths so that an astronomer can tell what elements are present in a star. However, if the star is receding (or approaching) these lines will be shifted to longer (or shorter) wavelengths by the Doppler effect. If the star is rotating, a line is broadened from its normal condition because some of the light comes from parts of the star which are moving away and some from parts which are approaching. From the amount of the broadening, conclusions may be drawn about the star's rotation. About 1930, Edwin Hubble of the Mount Wilson Observatory deduced from spectra of distant galaxies that their light was Doppler shifted to longer or redder wavelengths by an amount which increased with the distance of the galaxy. This red shift of the light from the galaxies indicated that they were rushing away from us at velocities which increased with their distance. This led to the idea of an expanding universe and by an extrapolation backwards in time to its origin from an initial explosion or "big bang" from which it has been expanding ever since. Summary: The Doppler effect is useful for determining the velocity of approach or recession of moving objects. Wavelengths are stretched out or longer when a wave-producing object is receding. Wavelengths are compressed or shorter when a wave-producing object is approaching. The wavelength of light from distant galaxies is increased making it redder. This red shift of the light indicates that the galaxies are receding. B. 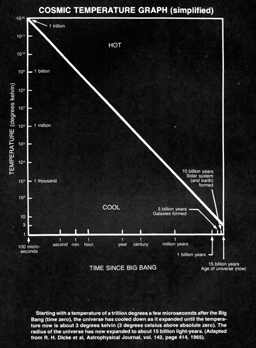The Big Bang Background (3 degrees) As discussed in the previous section on the Doppler effect, the discovery that the universe was expanding led to the idea that in the distant past the entire universe was in a super compact (high density) state and that its expansion began abruptly with an enormous explosion popularly called the "big bang". In the 1930s and 40s George Gamow and others outlined a history of the universe: Beginning with the big bang, a fireball of intense radiation blasted outward at the speed of light. Initially this primordial fireball had a temperature of billions of degrees. As it expanded it cooled down. Refinements of Gamow's original idea have led to present theories that put the temperature of the primordial fireball at the time of the explosion (time zero) at a trillion degrees or more. Cooling was rapid at first, decreasing billions of degrees in a matter of seconds. With passage of time, cooling became more gradual. After about 15 billion years (or now), it has reached a value of about 3 degrees celsius above absolute zero as determined by Arno Penzias and Robert Wilson of the Bell Telephone Laboratories in 1965 (see COSMIC SEARCH for March 1979, Vol. 1, No. 2, page 32). The change in the temperature of the universe with time is shown in the accompanying Cosmic Temperature Graph. The present age of the universe is taken as 15 billion years, with galaxies forming 5 billion years after the big bang (time zero) and the solar system (with the earth) forming 10 billion years after time zero (see the Cosmic Calendar). The 3 degree sky background which Penzias and Wilson measured with their radio telescope at a wavelength of 7.4 centimeters establishes a limit to the sensitivity of radio telescopes as discussed in ABCs in the March 1979 issue of COSMIC SEARCH. This 3 degree background temperature covers the entire sky. We have evolved from cinders of the fireball. As we now look skyward we see the cooled remnant of the primordial fireball in every direction. From this fireball the whole universe, as we know it, has evolved. We are, like a phoenix, creatures born of a fireball. Summary: The universe began with the explosion of a primordial fireball (the "big bang") about 15 billion years ago. Galaxies formed about 10 billion years ago. The solar system (with the earth) formed about 5 billion years ago. At the time of the big bang the fireball temperature was trillions of degrees. Now, 15 billion years later, the temperature of the universe (or remnant of the fireball) is 3 degrees celsius above absolute zero. C. 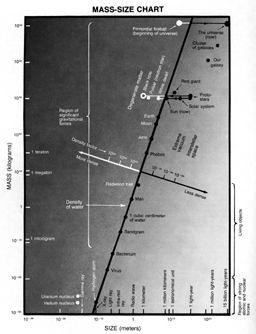All Things both Great and Small: A Chart for Everything in the Universe [Caption (in bold) for Mass-Size Chart below]     A chart for everything in the universe from a tiny atomic nucleus to the largest galaxy to the universe itself.     Each point indicates the mass of an object (in kilograms) and its size (in meters). The (average) density of the object is also indicated in terms of the density of water (heavy slant line), densities being greater to the left of this line and less to the right.     Note that the universe during its evolution progressed from a high density to a low density while a gas and dust cloud (protostar) during its evolution into a star and finally a black hole progresses from a low density to a very high density. (Adapted from J. D. Kraus, "Radio Astronomy", McGraw-Hill, 1966). Everything in the universe from enormous galaxies to tiny atoms has a size, which can be expressed as a maximum length or diameter and a mass. The accompanying Mass-Size displays objects from a helium nucleus to clusters of galaxies and the universe itself, each point on the chart indicating the mass of an object (in kilograms) and its size (in meters). On the assumption that the object is spherical or cubical, the size dimension is indicative of its volume. The ratio of the mass to the volume of an object is a measure of its density. Thus, a cubic meter of air has a mass of about one kilogram but a cubic meter of lead has a mass 10,000 times as much, so, relatively speaking, air is said to have a low density while lead has a high density. Water is intermediate with a density about 800 times that of air but one eleventh that of lead. From the position of an object on the chart its (average) density can be inferred. Thus, the chart also serves as a Density Diagram for everything in the universe. Objects on the slanting line have the density of water with densities increasing to the left of the line and decreasing to the right. Atomic nuclei and degenerate matter in the form of neutron stars and black holes have the greatest density of anything in the universe while galaxies or the universe itself have the lowest densities, being equivalent on the average to an extreme vacuum. There is a tendency for large objects to have a lower density and small objects a higher density. The earth and living objects are in between with densities near that of water. The region of stars and planets, which is of particular interest to SETI, occupies only a small part of the diagram. The figure is constructed with logarithmic scales so that the enormous ranges of mass, size and density are greatly compressed. Summary: Black holes have enormous densities. A pulsar (neutron star) is almost as dense, a thimble full weighing as much as 10,000 Empire State Buildings. Galaxies have low densities. The earth and living objects are in between. Cosmic Calendar 15 billion BC Universe began (BIG BANG) 10 billion BC Our galaxy formed 5 billion BC Solar system (sun, earth and other planets) formed 2 million BC Homo sapiens emerged 5000 BC Writing invented 1888 AD Hertz produced radio waves 1903 AD Letter "S" sent by radio waves across Atlantic Ocean by Marconi 1959 AD Cocconi and Morrison proposed SETI 1960 AD First attempt to detect extraterrestrial civilizations by Drake 1979 AD First issue of COSMIC SEARCH Distance Table Distances in light travel time (approx.) Earth to moon 1 second Earth to sun 500 seconds (8 min.) Sun to Mars 12.5 minutes Sun to Jupiter 40 minutes Sun to Pluto 5.5 hours Solar system diameter (at orbit of Pluto) 11 hours Sun to nearest star 4 years Sun to center of galaxy 30,000 years Diameter of galaxy 100,000 years Distance of Andromeda galaxy 2 million years Distance to "edge" of universe 15 billion years To convert light travel time to kilometers multiply travel time in seconds by velocity of light (300,000 kilometers per second).